Talk:Angular momentum
| This It is of interest to the following WikiProjects: | |||||||||||
‹See TfM›
| |||||||||||
| This article was nominated for merging with Introduction to angular momentum on July 2010. The result of the discussion was no consensus. |
Re "Electrons and photons need not have integer-based values for total angular momentum, but can also have fractional values.[29]"
[edit]I checked the reference. This is its abstract:
"The angular momentum of light plays an important role in many areas, from optical trapping to quantum information. In the usual three-dimensional setting, the angular momentum quantum numbers of the photon are integers, in units of the Planck constant ħ. We show that, in reduced dimensions, photons can have a half-integer total angular momentum. We identify a new form of total angular momentum, carried by beams of light, comprising an unequal mixture of spin and orbital contributions. We demonstrate the half-integer quantization of this total angular momentum using noise measurements. We conclude that for light, as is known for electrons, reduced dimensionality allows new forms of quantization."
This is a very specific thing to a very specialized case: reduced dimensions. Yet the [wikipedia] article doesn't allude to that, and is speaking instead in the context of spin-orbit interactions. That makes it misleading. It is not mentioned in the article and does not apply to the situation given. I suggest it be deleted. Betaneptune (talk) 00:27, 2 May 2019 (UTC)
Inclusion of radians in angular momentum units
[edit]The idea of angles being dimensionless is arguable Dimensional_analysis#Siano's_extension:_orientational_analysis, but assuming angles are in fact dimensionless, I think we need to specify which angular measure is being used. As a simpler example, consider angular velocity. Is it measured in radians/sec, degrees/sec, cycles/sec? Just because angles are dimensionless, the measuring stick used to measure angles needs to be specified. Given a pure number as the angular velocity, shouldn't one be able to take the units of measurement and determine the actual angular velocity? The SI base units are not equivalent to the dimensions of angular velocity, they are a means of turning a pure number into an actual angular velocity, and you cannot do that without specifying the measure of angle, dimensionless or not.PAR (talk) 23:43, 11 May 2019 (UTC)
- dimensionless is not the same as unitless. Radians are a ratio: (length this way)/(length that way). Length cancels out so there is no dimension to it, but you are left with (this way)/(that way) which is a unit (very odd unit). AManWithNoPlan (talk) 00:34, 12 May 2019 (UTC)
- if dimensionless = unitless, then radians = gradians = numbers =... which is obviously rubbish AManWithNoPlan (talk) 00:36, 12 May 2019 (UTC)
- Dimension of the angle measure is a valid topic for dispute. But this article is titled “angular momentum”, goddamn, not “angular velocity”. If nobody is going to clarify which angle must one draw to determine the angular momentum, then I side with Dolphin51 against this poorly considered addition. Incnis Mrsi (talk) 11:51, 12 May 2019 (UTC)
- Wikipedia publishes what has already been published in reliable sources. That way, readers can independently verify what they read in Wikipedia. Our objective must now be to determine what units have been published for angular momentum in reliable sources. If both with, and without, the “per radian” have been published we must attempt to give due weight to the majority view of those reliable sources.
- I am looking forward to investigating exactly what the sources on my bookshelf say on this matter, but unfortunately I won’t be at home to do so for about three days. However, I will do so.
- In the interim, I invite all others with an interest in this matter to investigate the sources available to them, and let us know on this Talk page. Dolphin (t) 12:20, 12 May 2019 (UTC)
To make this radian thing work, an anon made an uncommented addition of rad^{-2} in Moment of inertia (which I reverted as vandalism, which might be an over-reaction). The nice thing about radians is not just that they are dimensionless, but also that they are "natural", that is, a radian is one meter of motion at one meter of radius, for example. If you want to have radians in angular velocity, and use moment of inertia times angular velocity as angular momentum, then yes you also want radians^{-2} in your moment of inertia units. But I don't find any sources that do it that way, since, as the article says, "for a point mass the moment of inertia is just the mass times the square of the perpendicular distance to the rotation axis"; there's no reason to introduce angles. They just treat the natural dimensionless radian as unity instead, when multiplying by an angular velocity (units s^{-1}). Dicklyon (talk) 17:41, 12 May 2019 (UTC)
- A point mass circling the origin at distance r meters, velocity v meters per second, has an angular velocity of ω=v/r radians/sec = 180 v/(π r) degrees/sec = v/(2π r) cycles/sec. It has a moment of inertia of I=m r2 (kg m2) and an angular momentum of L=Iω:
- L= m r v (kg m2 radians/sec)
- L= 180 m r v/π (kg m2 degrees/sec)
- L= m r v/(2π) (kg m2 cycles/sec)
- We can summarize by saying ω=v φ/r where φ is a conversion factor between your chosen angle unit and radians and has units equal to that angle unit.
- An angle is not a dimensionless physical constant. A dimensionless physical constant never changes its value and so there is no need to assign it "dimensionless units" or some such thing. Siano notes that if you have what you presume to be a valid physical equation and it contains a term Sin(θ)+Cos(θ), then your presumption is wrong. Its the "this way/that way" problem that AManWithNoPlan mentioned above. The above term is like (this way/that way+that way/that way). Siano says we should view the angle θ as having dimensions such that θ2n is dimensionless and θ2n+1 has dimensions θ. Clearly then, the Taylor expansion of Sin(θ) has dimension θ and the Taylor expansion of Cos(θ) is dimensionless, which allows you to reject the above presumption. You can develop a logically consistent theory which is more useful than saying θ is dimensionless (e.g. the above example). You can call it ad hoc if you want, but as far as I'm concerned, quantum mechanics is ad hoc in the same sense: Extremely logically consistent and extremely useful, and if it doesn't give you a comfortable intuitive understanding, you don't discard it, you go to work.
- I don't want to introduce Siano's development into this article, but the idea that angular velocity (and angular momentum) need make no reference to the units of angular displacement is wrong and not helpful. That said, I agree with Dolphin51 that strong consideration should be given to common usage, while avoiding rote parroting. PAR (talk) 04:21, 13 May 2019 (UTC)
- That Siano approach looks pretty interesting, but does anyone use it when talking about moment of inertia and angular momentum? As far as I know, they don't don't; effectively using your conversion factor φ = 1, or implicitly radians, but not needing to say so since "natural" angular units are generally understood in these things. Bring us some book sources if this is not so. Dicklyon (talk) 04:30, 13 May 2019 (UTC)
- I've never seen Sianos "directional analysis" used except in a few papers on dimensional analysis, and that's why it should not be mentioned in this article. It's just that I like to take things that were pounded into my head as a freshman, check for flaws under a bright light, and pound them back in if they appear flawless. This is not the case with "angles are dimensionless", and Siano does the best job of pointing that out. I think the multiple ways of writing angular momentum that I mentioned above also point out the flaws. They are all valid, and since the idea of a "dimensionless unit" sounds like an oxymoron, I would conclude that angles have dimension. But ok, lets go with the dimensionless angle idea since it is so prevalent, but the idea that you can ignore the "dimensionless units" of angular displacement is just wrong. Radians should be included. PAR (talk) 04:51, 13 May 2019 (UTC)
- I don't think we're going to take your word for it. Tell us who pounded this into your head and maybe we can investigate. The exact same problem comes up, by the way in Nepers, which the SI defines as a dimensionless unit of value unity. Nepers and radians are the real and imaginary parts of complex natural logarithms, which have no "physical" dimension. Dicklyon (talk) 04:58, 13 May 2019 (UTC)
- "Pounded into my head" was not a precise statement that I can reference, sorry if I was winging it, but my experience was that I learned "angles are dimensionless" as received wisdom rather than a proof. Isn't that how you learned it? If not, what was the justification provided for that idea?
- I don't think we're going to take your word for it. Tell us who pounded this into your head and maybe we can investigate. The exact same problem comes up, by the way in Nepers, which the SI defines as a dimensionless unit of value unity. Nepers and radians are the real and imaginary parts of complex natural logarithms, which have no "physical" dimension. Dicklyon (talk) 04:58, 13 May 2019 (UTC)
- I've never seen Sianos "directional analysis" used except in a few papers on dimensional analysis, and that's why it should not be mentioned in this article. It's just that I like to take things that were pounded into my head as a freshman, check for flaws under a bright light, and pound them back in if they appear flawless. This is not the case with "angles are dimensionless", and Siano does the best job of pointing that out. I think the multiple ways of writing angular momentum that I mentioned above also point out the flaws. They are all valid, and since the idea of a "dimensionless unit" sounds like an oxymoron, I would conclude that angles have dimension. But ok, lets go with the dimensionless angle idea since it is so prevalent, but the idea that you can ignore the "dimensionless units" of angular displacement is just wrong. Radians should be included. PAR (talk) 04:51, 13 May 2019 (UTC)
- That Siano approach looks pretty interesting, but does anyone use it when talking about moment of inertia and angular momentum? As far as I know, they don't don't; effectively using your conversion factor φ = 1, or implicitly radians, but not needing to say so since "natural" angular units are generally understood in these things. Bring us some book sources if this is not so. Dicklyon (talk) 04:30, 13 May 2019 (UTC)
- I am not clear on what your preference would be for this article. Would you prefer that radians be considered a dimensionless unit of value unity and that they not be mentioned in the "SI units" entry? Looking at the International System of Units article, I would support my point by noting that the SI units for angular velocity is radians/sec, and that therefore the units of angular momentum are kg m2 rad/sec, since moment of inertia has SI dimensions kg m2.
- It's interesting about Nepers and radians, I didn't know that. Siano notes that Exp(θ) is never found in a physical equation, while Exp(i θ) is ubiquitous. The imaginary unit i has dimensions of θ rendering i θ dimensionless. (Real numbers are dimensionless "this way" and i has dimensions of θ "that way" in the complex plane). Strictly speaking, the only physical expression involving a logarithm must be of the form ln(r Exp(i θ)/ro] where r and ro are dimensionally equal. Then ln(r Exp(i θ)/ro]=ln[|r/ro|]+i(θ+2nπ) which is clearly dimensionless. It seems to me that in Siano's development, Nepers are superflous as a unit, (i.e., a "dimensionless unit"), while radians are not. Nevertheless, if SI says its a unit, we cannot ignore that. ln(θ] is likewise never found in a physical equation.
- “A point mass circling the origin” – what can it prove? It makes sense for point-like particles (and, more generally, for a kind of physical body, in any case non-relativistic as otherwise p = mv isn’t true), but this metaphor fails miserably for fields. What is the general formula? It is L = r × p. To me, the English term “angular momentum” is a misnomer; the thing is actually “rotational momentum”. Incnis Mrsi (talk) 05:34, 13 May 2019 (UTC)
- I'm not sure what your point is, except for the misnomer. You may be right, but as long as we use the same words for the same meanings, we can communicate, which is most important. PAR (talk) 05:48, 13 May 2019 (UTC)
- Again, not all physical bodies behave in the Newtonian p = mv fashion. Momentum can’t be reduced to velocity, it can’t be compared to the positional vector in the same sense as velocity; they don’t form any angle together and you must use cross product instead of drawing φ = const rays or triangles. Incnis Mrsi (talk) 05:58, 13 May 2019 (UTC)
- I'm not sure what your point is, except for the misnomer. You may be right, but as long as we use the same words for the same meanings, we can communicate, which is most important. PAR (talk) 05:48, 13 May 2019 (UTC)
- Cross product states: "The cross product is defined by the formula axb=||a|| ||b|| sin(θ) where θ is the angle between a and b in the plane containing them.". Newtonian mechanics (p = mv with m constant) is a special case of the standard model which involves quantum and relativity. It's not universally valid, and the "derivation from other quantities" in the box is either incorrect or the article is limited in scope. But, importantly for this discussion, the units are not a special case and the question is "do we include radians in the SI units portion of the box". My contention is yes, because the SI units for angular velocity is radians/sec. PAR (talk) 11:05, 13 May 2019 (UTC)
- Sure, I know what the cross product is – the sine function maps angles to real numbers from [−1, 1]. How one measures angles between two vectors—in radians, degrees, or BAMS—is irrelevant to dimension of its value, which is merely the product of dimensions of arguments. What now? L = r × p is almost universal… valid everywhere we can neglect intrinsic angular momenta. The moving point particles example has narrower applicability; see above. Suppose we apply dimensional—or directional—analysis to the rotational momentum. Why should we think about point masses and their velocities then? Incnis Mrsi (talk) 12:26, 13 May 2019 (UTC)
- Ok, so you are saying that since Sin[θ] is dimensionless, there are no radians to be found in the units of angular momentum? And therefore L=Iω means moment of inertia must have 1/radians in its dimensions? Just trying to clarify.PAR (talk) 13:16, 13 May 2019 (UTC)
- I won’t object against rad−1 in the dimension of moment of inertia. But the dispute may be compared with a question of the physical dimension of 4-vectors in relativity. Is 4-displacement m or s? When we go along a world line, it arguably should be s (as the differential of proper time), but in general? Whatever. Is 4-momentum kg m s−1 (momentum) or kg m2 s−2 (energy)? For massive bodies it is convenient to think of its absolute value as of energy, and the same for p0, but on the other hand p1, p2, p3 constitute the 3-momentum p in the respective reference frame. Whereas—in general—either of two dimensions as they are equivalent. Quibbling over rad = 1 in mechanics amounts to quibbling over c = 1 in relativity. Incnis Mrsi (talk) 15:24, 13 May 2019 (UTC)
- Ok, so you are saying that since Sin[θ] is dimensionless, there are no radians to be found in the units of angular momentum? And therefore L=Iω means moment of inertia must have 1/radians in its dimensions? Just trying to clarify.PAR (talk) 13:16, 13 May 2019 (UTC)
- If you say the momentum 4-vector is (E/c,px,py,pz), that's kg m/sec. If you want to define a different vector (E,px/c,py/c,pz/c), that's a different vector. What name tag you want to put on the two vectors is uninteresting, as long as we agree on the naming. I would call the first the momentum 4-vector because it has units of momentum. I agree, the rad=1 question is analogous to the c=1 question. c is the ratio of an increment in the space direction (this way) divided by an increment in the time direction (that way), and if we choose, say, meters as the measure of space and time, then c=1, but this irons over the problems that directional analysis presumes to solve. Dimensionally, c=1 times this way/that way. Just like radians in space. Ok, directional analysis may be logically consistent, useful, but it's not mainstream, so we can't use it here. All I'm saying is that we can use an understanding of directional analysis when dealing with the troubles that arise when we ignore it, while not using it explicitly. With respect to this article, the trouble is arbitrarily adding 1/rad to the moment of inertia just to make L=Iω dimensionally well-behaved, when there is no reason to do so based on the definition of moment of inertia (I). It also relates to the pseudovector problem. Pseudovectors (Like rxp) are not independent of the hand-rule. If whoever invented the right hand rule had specified a left hand rule, all our pseudovectors (like L) would be reversed in sign. Directional analysis explains that by saying Sin[θ] has dimensions of θ, so pseudovectors have the dimensions of the product of the dimensions of r and p and θ That means you will never find a physical equation of the form a.b + cxd where a,b,c,d are physical (non-pseudo) vectors. Likewise, you will never find a physical equation involving Sin(θ)+Cos[θ), or Exp(θ), etc. These facts follow directly from directional analysis, but "angles are dimensionless" provides no clue. PAR (talk) 19:29, 13 May 2019 (UTC)
Bottom line
[edit]I've added this as a summary if we wish to submit this as an RFC. PAR (talk) 01:25, 14 May 2019 (UTC)
The problem is this:
- 1) Angular velocity (ω) has SI units of rad/sec. (this has been changed to (dimensonless radians)/sec as per SI PAR (talk) 18:56, 15 May 2019 (UTC))
- 2) Moment of inertia (I) has units of kg m2
- 3) Angular momentum = L = I ω
- 4) Angular momentum = L = r x p (radius cross linear momentum)
Declaring angles to be dimensionless:
- 3) implies that the units of angular momentum are kg m2rad/sec
- 4) implies that the units of angular momentum are kg m2/sec
Orientational analysis resolves this problem, but it is perhaps not familiar or common enough to be used in this article. Often, the literature appears to simply ignore the problem.
The question is: What units should the Wikipedia article on angular momentum specify for angular momentum?
Orientational analysis References:
- Page, Chester H. (1961), "Physical Entities and Mathematical Representation" (PDF), Journal of Research of the National Bureau of Standards - B. Mathematics and Mathematical Physics, 65B (4): 231
{{citation}}: CS1 maint: ref duplicates default (link) - Siano, Donald (1985), "Orientational Analysis – A Supplement to Dimensional Analysis – I", Journal of the Franklin Institute, 320 (6): 267–283, doi:10.1016/0016-0032(85)90031-6
- Siano, Donald (1985), "Orientational Analysis, Tensor Analysis and The Group Properties of the SI Supplementary Units – II", Journal of the Franklin Institute, 320 (6): 285–302, doi:10.1016/0016-0032(85)90032-8
- Araneda, J. (1996), "Dimensional-directional analysis by a quaternionic representation of physical quantities", Journal of the Franklin Institute, 333: 113–126, doi:10.1016/0016-0032(96)85843-1
{{citation}}: CS1 maint: ref duplicates default (link)
- deBoer, Jan (1979), "Group properties of quantities and units", American Journal of Physics, 47: 818
{{citation}}: CS1 maint: ref duplicates default (link)
NOT PEER REVIEWED, but useful:
https://www.preprints.org/manuscript/201810.0525/v2/download
http://www-personal.umich.edu/~dsbaero/library/DSBCSMPapers/DimensionalAnalysisHarish.pdf
http://paulkienitz.net/future/torque.html
Discussion of Bottom line
[edit]- I think the two points of view contrasted in the deBoer paper get used in a mixed up way without realizing the distinction. But basically the SI says that's OK, just treat radian as 1. So including the radians in angular velocity, while omitting it from angular momentum and moment of inertia, as is conventional, is a non-problem. Possibly worth a footnote. Dicklyon (talk) 04:54, 15 May 2019 (UTC)
- For someone trying to analyze a problem using dimensional analysis, which demands dimensional homogeneity, it is a big problem. Also, SI said (fify years ago) that it could not decide between the two points of view. So that made me wonder what the situation is now. According to https://www.nist.gov/pml/special-publication-811/nist-guide-si-chapter-4-two-classes-si-units-and-si-prefixes, they have made a decision, and, in base units, angular velocity is measured in sec-1, so yes, as you say, treat radian as 1, but in the L=Iω equation, ω is restricted to being expressed in (dimensionless radians)/sec. (I have removed the "unarguable" from 1) above, even tho it's still true). This formally resolves the problem, and I have reverted my own addition of rad to the units, and added some clarifications, including a link to orientational analysis, for those interested. I'm not happy with the situation, but criticizing SI is OR, and I would be less happy with that.
- There's an interesting article by Chester Page at https://aapt.scitation.org/doi/10.1119/1.11704 entitled "Rebuttal to deBoer's "Group properties of quantities and units"". It consists of three paragraphs. I don't understand the first two, but the third is interesting. (I think he meant to say "problems with" rather than "simplicity of"). PAR (talk) 07:33, 15 May 2019 (UTC)
- Fascinating. And yes I sympathize with the problem. But, OR, as you say. Dicklyon (talk) 03:24, 16 May 2019 (UTC)
- There's an interesting article by Chester Page at https://aapt.scitation.org/doi/10.1119/1.11704 entitled "Rebuttal to deBoer's "Group properties of quantities and units"". It consists of three paragraphs. I don't understand the first two, but the third is interesting. (I think he meant to say "problems with" rather than "simplicity of"). PAR (talk) 07:33, 15 May 2019 (UTC)
- It occurs to me that if radians are dimensionless, so are degrees. That would mean than L=Iω is valid for any unit of angular measurement, as long as the units are consistent on either side. In other words, one would say units of L are ML2θ/T, the units of ω are θ/T, where θ is the dimensionless unit of angle (radians, degrees, etc. whatever) and since it is dimensionless, it is dropped in the SI expression of the units for L and ω. Would anyone object to the article expressing this explanation? PAR (talk) 03:09, 19 May 2019 (UTC)
- Is there anything like that in sources? Dicklyon (talk) 03:19, 19 May 2019 (UTC)
- It occurs to me that if radians are dimensionless, so are degrees. That would mean than L=Iω is valid for any unit of angular measurement, as long as the units are consistent on either side. In other words, one would say units of L are ML2θ/T, the units of ω are θ/T, where θ is the dimensionless unit of angle (radians, degrees, etc. whatever) and since it is dimensionless, it is dropped in the SI expression of the units for L and ω. Would anyone object to the article expressing this explanation? PAR (talk) 03:09, 19 May 2019 (UTC)
- Ok, I apologize in advance for the long reply. I understand that this seems like a lot of nit-picky stuff, but I am trying to write a Mathematica program that performs dimensional analysis along the lines of Buckingham_π_theorem#Formal_proof which also incorporates the advantages of orientational analysis (see also the SCADE[1] reference which describes enhanced dimensional analysis on computer programs and which would have prevented the 1999 screwup that destroyed the Mars Climate orbiter). These questions then go from nitpicky to vitally important. I'm just trying to improve the Wikipedia articles using these insights.
References
- ^ Schlick, R.; Le Sergent, T. (2006). "Checking SCADE Models for Correct Usage of Physical Units". Computer Safety, Reliability, and Security. 4166. Berlin: Springer. Retrieved 19 May 2019.
- Your question led me to look for sources, and so far, the short answer is no, so cancel that idea. The problem remains that when to use "rad" and when not to is in a confused state, with NIST and SI not quite on the same page.
- Looking at https://www.nist.gov/pml/special-publication-811/nist-guide-si-chapter-4-two-classes-si-units-and-si-prefixes, angular velocity is specified as having the "symbol" of rad/s, while the "Expression in terms of SI base units" says m·m-1·s-1 = s-1. From this I gather that s-1 is the proper thing to enter in the infobox under "In SI base units". I'm wondering what is meant by "Symbol", however.
- Also on the above page, the following paragraph: (Italics are mine)
A derived unit can often be expressed in several different ways through the use of base units and derived units with special names. In practice, with certain quantities, preference is given to using certain units with special names, or combinations of units, to facilitate the distinction between quantities whose values have identical expressions in terms of SI base units. For example, the SI unit of frequency is specified as the hertz (Hz) rather than the reciprocal second (s-1), and the SI unit of moment of force or torque is specified as the newton meter (N · m) rather than the joule (J).
- Then there is a statement in the torque article that says:
From the official SI website: "...For example, the quantity torque may be thought of as the cross product of force and distance, suggesting the unit newton metre, or it may be thought of as energy per angle, suggesting the unit joule per radian."
- While the problem and its solution is clear, it is difficult to shoehorn these insights into Wikipedia while (necessarily and rightly) remaining in bounds.
Should Iω stand before r × p?
[edit]By the way, is it right to start the quantitative treatment from “L = Iω”? To me, it is a thoughtless replication of “p = mv”, but the analogy is flawed. While we can explain what the mass is without referring to the momentum directly—and mass is a scalar, after all—it’s impossible to define the moment of inertia without defining the rotational momentum first (either explicitly, or via e.g. torque).
Moreover, we don’t live in the 19th century. Modern physics knows that such things as a particle with spin or a p-orbital rotate without any angular velocity. The same for fields, even such classical field theories as electrodynamics. Incnis Mrsi (talk) 05:17, 13 May 2019 (UTC)
- No, the moment of inertia is defined without reference to angular momentum, it is strictly a function of the relative positions of the particles, or the density distribution. I agree with your point about quantum fields and particles, and L = Iω is not the way to transition to the QM theory, and moment of inertia is, I guess, not as simple.PAR (talk) 05:54, 13 May 2019 (UTC)
- “Strictly a function of the relative positions of the particles” makes sense for a “strictly” rigid body only. Incnis Mrsi (talk) 06:06, 13 May 2019 (UTC)
- I agree. PAR (talk) 10:27, 13 May 2019 (UTC)
- But the scope of the angular momentum is immensely broader, even in Newtonian physics (think celestial mechanics). Indeed the rigid example is an important example, whereas the article—including the photo at the top— currently suggests that it’s the main domain where this notion may be applied. Incnis Mrsi (talk) 12:09, 13 May 2019 (UTC)
- Again I agree. As such, the article concentrates on introductory concepts, but does not do the full development.PAR (talk) 19:46, 13 May 2019 (UTC)
- But the scope of the angular momentum is immensely broader, even in Newtonian physics (think celestial mechanics). Indeed the rigid example is an important example, whereas the article—including the photo at the top— currently suggests that it’s the main domain where this notion may be applied. Incnis Mrsi (talk) 12:09, 13 May 2019 (UTC)
- I agree. PAR (talk) 10:27, 13 May 2019 (UTC)
- “Strictly a function of the relative positions of the particles” makes sense for a “strictly” rigid body only. Incnis Mrsi (talk) 06:06, 13 May 2019 (UTC)
Revision of last paragraph of Introduction
[edit]I revised the wording of the last paragraph of the introduction and this has been reverted almost immediately as being a 'bad' edit so I will explain my changes. Firstly, angular momentum is a physical quantity, and so calling it an 'operator' is slightly iffy - an operator is used to represent it in the mathematical formalism of quantum mechanics. That doesn't mean that angular momentum is literally an operator, which is purely a mathematical concept. i.e. it is convenient for us humans to use operator formalism to explain angular momentum, that doesn't mean that any physical measurement will tell us that angular momentum is an operator. Again, the introduction then goes on to talk about eigenvalues - saying that angular momentum is quantized is enough, an eigenvalue reference conjures up associations with linear algebra again which is mathematical rather than physical. It then went on to say 'one projection (one component)' - we don't need two words for one thing in the introduction, especially with brackets. It then commits the same offence in saying 'a particle (i.e. an excitation of a quantum field) - again too much information. It then says 'for technical reasons' particles still possess angular momentum. What are these technical reasons exactly? It is simply an observation that particles possess spin, there is no technicality to it. The person who revised my introduction complained that I 'pushed' that 'quantum = fundamental', this being problematical. What I did was replace the phrase 'in quantum mechanics' with 'at a more fundamental level'. Again, it's silly to say 'in quantum mechanics blah blah blah', just as it's silly to say 'in classical mechanics, a spring stretches'. The primary thing we are dealing with here is nature, not mathematical representations of things. Quantum Mechanics is a formalism used to represent phenomena that are actually observed, so we should talk primarily of the phenomena, without overly stressing the mathematical theory, especially in the introduction. I did make an error however of perhaps implying that all particles are spin-1 by talking of three components of angular momentum at a quantum level. I have removed that now. As such, I have reverted to my previous edit. Oward98 (talk) 08:55, 7 July 2019 (UTC)
- You replaced properly sourced content with unsourced content. And see also wp:edit warring and wp:BRD. - DVdm (talk) 08:59, 7 July 2019 (UTC)
- Oward98 may disagree on such details as whether the angular momentum “is” an operator, but pushing almost the same text three times and dismissing criticism of that text is a no-go. Incnis Mrsi (talk) 09:57, 7 July 2019 (UTC)
- Good edit, this. - DVdm (talk) 10:20, 7 July 2019 (UTC)
Proposed move of redirect for angular velocity to angular momentum
[edit]Edit: FALSE ALARM. I hadn't noticed that page angular velocity already exists, given that rotational velocity redirected to rotational speed instead. I fixed the redirect to point to angular velocity with a for hatnote at rotational speed.
See Talk:Rotational_speed#Proposed_move_of_redirect_for_angular_velocity_to_angular_momentum
— MaxEnt 21:49, 22 April 2020 (UTC)
- On the way by, I also changed a creaky redirect from angular force to centripetal force (yikes!) to point instead to torque so now I feel at least partially redeemed. — MaxEnt 22:27, 22 April 2020 (UTC)
Gyroscopic refusniks
[edit]- Chris Lee: Atoms spin backward while flying along a surface — 23 September 2019
Thought experiment: if I take two parallel metal plates—both circular of the same diameter—and between these I mount six spinning tops on jeweled bearings in a hexagon pattern, and then I rotate the plate apparatus around it's geometric center, the tops themselves should exhibit gyroscopic stability.
But what if the tops themselves are actual atoms? Do the individual atoms remain gyro-stable and as such their electronic and nuclear mass contributing nothing much to the angular inertia for the object in question?
I thought this must be a dumb question, but Chris Lee above shows it maybe isn't as dumb as I thought. — MaxEnt 22:16, 22 April 2020 (UTC)
- Here we can only discuss the article, not the subject—see wp:Talk page guidelines. You can ask questins at our wp:Reference desk/Science. - DVdm (talk) 06:33, 23 April 2020 (UTC)
- By the way, user MaxEnt, please sign all your talk page messages with four tildes (~~~~), not three (~~~). With four tildes your user talk page shows as a link in your signature — see Help:Using talk pages. Thanks. DVdm (talk) 06:39, 23 April 2020 (UTC)
Substandard animation
[edit]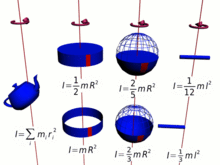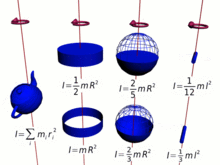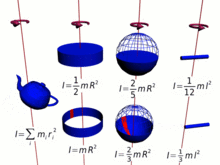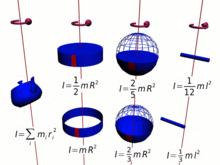
On Commons, there are now two talk page remarks (one is my own) concerning deficiencies of this animated illustration. — MaxEnt 17:31, 12 June 2021 (UTC)
Article's Intro Should Be Simplified
[edit]If you don’t know anything about this, trying to read the article is difficult. The initial paragraph should be modified so it is meaningful to someone who is not oriented with any of the acronyms or science referenced.Jimerb (talk) 11:21, 19 September 2021 (UTC)
- @Jimerb: Fair comment. A possible explanation as to why the lead to this article is not reader-friendly is that we used to have an "Introductory" article to help readers new to this topic ease into the science of angular momentum. It is no longer available in mainspace but it is still there for those who know where to look. Have a look at:
- Do you find this helpful? If there is some support for restoring this "Introductory" article to the mainspace and advertising its availability using a hatnote at the top of the current article, that can be done. Dolphin (t) 12:56, 19 September 2021 (UTC)
- @Dolphin51: Not really. The introductory article you referenced starts with a heavy duty formula in the third sentence. The article should start with a basic English understanding of what it is. Later on the article can get as complex as necessary but for someone who is trying to grasp what's going on, it should be a rudimentary explanation up front.
For example, I understand from the beginning of the introductory article that a frisbee has angular momentum. But i still have no idea what it is. The initial paragraph should be described simple enough that my grandmother would understand what it is. Also it would make more sense if the very basic description was on the main article then linking to an introductory article. Jimerb (talk) 03:49, 2 October 2021 (UTC)
Non-conservation of angular momentum in general relativity
[edit]The fact that there's no general conservation law of angular momentum in general relativity is explained in the books by Misner, Thorne, Wheeler, and by Hawking & Ellis. These are two classics, and their statements are still valid today. I added these references to the appropriate section, which had a "citation needed". Someone then eliminated these references saying "unreliable sources". Are we joking? If those two classics are "unreliable", then what is? (This is one of the reasons why for me it won't be a great loss if Wikipedia closes. It's just a large-scale personal blog, not a scholarly work.) pglpm (talk) 18:17, 27 February 2024 (UTC)
- @Pmpgl: The "not a reliable source" comment referred to changes that were made after yours. Note the username mentioned in the edit summary: "Reverting edit(s) by FrancescoGaiani (talk) to rev. 1202790228 by Pmpgl: Not providing a reliable source (RW 16.1)" This summary was explaining that they were restoring the page TO the revision you had made, not reverting your edit.
- DVdm then restored a later version of the page to remove other unsourced edits by FrancescoGaiani, which had also introduced a large amount of unsourced content. My suspicion is that DVdm did not notice that you had made edits in between FrancescoGaiani's edits, and didn't realize that restoring the older version also undid your work: [1]. GorillaWarfare (she/her • talk) 19:32, 27 February 2024 (UTC)
- @Pmpgl and GorillaWarfare: Yes, with this restoring edit, I also undid Pmpg!'s contribution. Sorry for that. - DVdm (talk) 22:20, 27 February 2024 (UTC)
The math for the total angular momentum of a set of particles may be wrong
[edit]It says that the equation for total angular momentum is
(1)
But the Ri and Vi are position and velocity w.r.t the origin, not respect to the center of mass. So it should be
(1)
Can someone tell me if I am right? And if my fix is correct? Frisk-256 (talk) 00:20, 23 July 2024 (UTC)



